Molar volume is the space occupied by one mole of a substance. This term is used in chemistry to determine the molecular volume reflected in cubic meters per mol (m3 x mol-1). A mole is the unit contemplated by the International System of Units that allows to measure and express a certain amount of substance. The molar volume of an ideal gas under normal conditions (1 atmosphere pressure and 0°C temperature) is equal to 22.4 liters/mol.
The molar volume can be calculated in substances in liquid, solid and gaseous states. Eventually, these individual laws were combined into a single equation—the ideal gas law—that relates gas quantities for gases and is quite accurate for low pressures and moderate temperatures. We will consider the key developments in individual relationships , then put them together in the ideal gas law.
The moles to liters/liters to moles conversion is straightforward and is based on the fact that the ideal gas equation is a good approximation for many common gases at standard temperature and pressure. Thus, we can rearrange terms in the ideal gas equation and write them like this . In chemistry, it is very important to know the volume of substances used for scientific experiments that merit extreme precision. To calculate molarity, divide the number of moles of solute by the volume of the solution in liters. If you don't know the number of moles of solute but you know the mass, start by finding the molar mass of the solute, which is equal to all of the molar masses of each element in the solution added together.
Once you have the molar mass, multiply the number of grams of solute by 1 over the molar mass to convert the grams into moles. Finally, divide the number of moles by the volume of the solution to get the molarity. Molality is an intensive property of solutions, and it is calculated as the moles of a solute divided by the kilograms of the solvent.
Unlike molarity, which depends on the volume of the solution, molality depends only on the mass of the solvent. Since volume is subject to variation due to temperature and pressure, molarity also varies by temperature and pressure. In some cases, using weight is an advantage because mass does not vary with ambient conditions. For example, molality is used when working with a range of temperatures. As per Avagadro law, equal volume of gases at same temperature and pressure contain equal number of moles regardless of chemical nature of gases. However Avagadro law is valid only for ideal gases and not for real gases.
Again consider the reaction in equation 3 above. Suppose you used 30.0 mL of the reactant 3COH. First convert this volume into mass using density (g/mL), then convert grams to moles using the molecular weight. Again, include units and set up your calculation so that milliliters and grams cancel in the calculation leaving an answer that has units of moles. The ideal gas law formula states that pressure multiplied by volume is equal to moles times the universal gas constant times temperature. The behavior of gases can be described by several laws based on experimental observations of their properties.
The pressure of a given amount of gas is directly proportional to its absolute temperature, provided that the volume does not change (Amontons's law). The volume of a given gas sample is directly proportional to its absolute temperature at constant pressure (Charles's law). The volume of a given amount of gas is inversely proportional to its pressure when temperature is held constant (Boyle's law). Under the same conditions of temperature and pressure, equal volumes of all gases contain the same number of molecules (Avogadro's law). To calculate the molarity of a solution, the number of moles of solute must be divided by the total liters of solution produced.
If the amount of solute is given in grams, we must first calculate the number of moles of solute using the solute's molar mass, then calculate the molarity using the number of moles and total volume. Gases whose properties of P, V, and T are accurately described by the ideal gas law are said to exhibit ideal behavior or to approximate the traits of an ideal gas. An ideal gas is a hypothetical construct that may be used along with kinetic molecular theory to effectively explain the gas laws as will be described in a later module of this chapter. Although all the calculations presented in this module assume ideal behavior, this assumption is only reasonable for gases under conditions of relatively low pressure and high temperature. In the final module of this chapter, a modified gas law will be introduced that accounts for the non-ideal behavior observed for many gases at relatively high pressures and low temperatures.
It is important to note that the molarity is defined as moles of solute per liter of solution, not moles of solute per liter of solvent. This is because when you add a substance, perhaps a salt, to some volume of water, the volume of the resulting solution will be different than the original volume in some unpredictable way. To get around this problem chemists commonly make up their solutions in volumetric flasks. These are flasks that have a long neck with an etched line indicating the volume. The solute is added to the flask first and then water is added until the solution reaches the mark.
The flasks have very good calibration so volumes are commonly known to at least four significant figures. The units of molar concentration are moles per cubic decimeter. They are noted as mol/dm³ as well as M (pronounced "molar"). The molar concentration of solute is sometimes abbreviated by putting square brackets around the chemical formula of the solute, e.g., the concentration of hydroxide anions can be written as [OH⁻]. In many older books or articles, you can find different units of molar solutions – moles per liter (mol/l).
Remember that one cubic decimeter equals to one liter, so these two notations express the same numeric values. Where Z is the gas compressibility factor, which is a useful thermodynamic property for modifying the ideal gas law to account for behavior of real gases. The above equation is basically a simple equation of state .
In chemistry and physics a mole describes an amount of a substance in grams equal to its atomic mass. For example, one mole of aluminum has a mass of 13 grams since it has an atomic mass of 13. Also, one mole of a substance contains Avogadro's number of atoms, namely 6.02 times 10 to the power 23. The molarity, or concentration of a solution, equals the number of moles in the solution divided by its volume.
Conversion between moles, molarity and volume is performed frequently in science problems. To calculate the concentration in metric dimensions, with other temperature and pressure conditions the Ideal Gas Law comes in handy. The volume divided by the number of molecules represents the molar volume of the gas with a temperature and pressure . An aqueous solution consists of at least two components, the solvent and the solute .
Usually one wants to keep track of the amount of the solute dissolved in the solution. One could do by keeping track of the concentration by determining the mass of each component, but it is usually easier to measure liquids by volume instead of mass. To do this measure called molarity is commonly used. Molarity is defined as the number of moles of solute divided by the volume of the solution in liters. Both terms are used to express the concentration of a solution, but there is a significant difference between them.
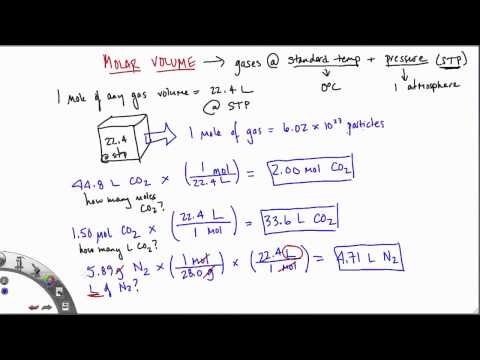
While molarity describes the amount of substance per unit volume of solution, molality defines the concentration as the amount of substance per unit mass of the solvent. In other words, molality is the number of moles of solute per kilogram of solvent . Is the volume occupied by one mole of a chemical element or a chemical compound. It can be calculated by dividing the molar mass by mass density (ρ). Molar gas volume is one mole of any gas at a specific temperature and pressure has a fixed volume. At standard temperature and pressure , 1 mole of ideal gas is equal to 22.4 liters.
Thus, the conversion ratio used in the formula below is 22.4. Avogadro was an Italian Physicist who first described the Avogadro constant as a hypothesis in 1811. He was trying to understand why in chemical reactions involving gases the observation that equal volumes of different gases had the same number of moles. This was found true even when the masses were very different.
The idea that a mole of any substance has exactly the same number of atoms no matter what the substance is made of was explained by Avogadro and his name has stuck to his number ever since. Two solutions that have the same molarity will have the same number of molecules of the chemical per liter but are likely to contain differing masses of that chemical per liter to achieve this. Whereas two solutions at the same concentration will have the same mass of the chemical per liter of solution but are therefore likely to have differing numbers of molecules of that chemical per liter. Provided some additional information is known, one value can be deduced from the other using the equations below. The concentration of a substance is the quantity of solute present in a given quantity of solution. Concentrations are usually expressed as molarity, the number of moles of solute in 1 L of solution.
We then convert the number of moles of solute to the corresponding mass of solute needed. To prepare a solution that contains a specified concentration of a substance, it is necessary to dissolve the desired number of moles of solute in enough solvent to give the desired final volume of solution. Figure 4.6 "Preparation of a Solution of Known Concentration Using a Solid Solute" illustrates this procedure for a solution of cobalt chloride dihydrate in ethanol. Note that the volume of the solvent is not specified. Because the solute occupies space in the solution, the volume of the solvent needed is almost always less than the desired volume of solution.
For example, if the desired volume were 1.00 L, it would be incorrect to add 1.00 L of water to 342 g of sucrose because that would produce more than 1.00 L of solution. As shown in Figure 4.7 "Preparation of 250 mL of a Solution of (NH", for some substances this effect can be significant, especially for concentrated solutions. Today's more and more there is an interest to express gas concentrations in metric units, i.e. µg/m3. Although expressing gaseous concentrations in µg/m3 units, has the advantage of metric expression, it has the disadvantage of being greatly influenced by changes in temperature and pressure. Additionally, because of difference in molecular weight, comparisons of concentrations of different gases are difficult. The SI base unit for the quantity of substance is the mole, which is abbreviated mol.
And liters are an SI unit of volume equal to one cubic decimeter. Now that you have the molar mass of the solute, you need to multiply the number of grams of solute in the solution by a conversion factor of 1 mole over the formula weight of the solute. This will give you the number of moles of the solute for this equation. The ideal gas equation contains five terms, the gas constant R and the variable properties P, V, n, and T. Specifying any four of these terms will permit use of the ideal gas law to calculate the fifth term as demonstrated in the following example exercises. In a mixture of ideal gases, the mole fraction can be expressed as the ratio of partial pressure to total pressure of the mixture.
This relationship between temperature and pressure is observed for any sample of gas confined to a constant volume. An example of experimental pressure-temperature data is shown for a sample of air under these conditions in Figure 9.11. One mole of water has a mass of 18 grams and volume of 18 milliliters or 0.018 liters.How much is a mole of water? A mole of water is Avogadro's number of water molecules. Avogadro's number is so large that it can be difficult to imagine its size. Finding the mass and volume of one mole of water is a great way to relate the units to something familiar.
Here is the calculation for the the mass and volume of one mole of water. For other substances in solid or liquid state, the molar volume is different and smaller than gaseous substances. One mole of any gas occupies the same volume when measured under the same conditions of temperature and pressure. In this experiment, the volume of one mole of hydrogen is calculated at room temperature and pressure. • To convert volume to moles, first convert to mass using density, then convert to moles using molecular weight. Again, be sure to include all units in your calculations.
You can get your moles by taking the molar mass of each of the elements in the solute and adding them together. Do it; the answer is in moles because the grams cancelled out.Then, go ahead and do your formula. The most common molar volume is the molar volume of an ideal gas at standard temperature and pressure (273 K and 1.00 atm). So you are not confused with similar chemical terms, keep in mind that molarity means exactly the same as molar concentration .
Molarity expresses the concentration of a solution. It is defined as the number of moles of a substance or solute, dissolved per liter of solution (not per liter of solvent!). Where x i is the mole fraction of the ith component, M i is the molecular mass of the ith component and ρmixture is the mixture density at the given temperature and pressure. The interest stems from that accurate measurements of the unit cell volume, atomic weight and mass density of a pure crystalline solid provide a direct determination of the Avogadro constant. An alternative way to define the concentration of a solution is molality, abbreviated m.
Molality is defined as the number of moles of solute in 1 kg of solvent. Would you expect a 1 M solution of sucrose to be more or less concentrated than a 1 m solution of sucrose? Molecules are composed of several atoms, for example a carbon dioxide molecule is made up of 1 carbon atom and 2 oxygen atoms. The molecular weight (or molecular mass or relative molecular mass ) is the sum of the atomic weights of all the atoms in the molecule.
Thus, the amount of substance in moles is equal to the volume of ideal gas in liters divided by the conversion ratio of 22.4 L/mol. Unlike converting the mass in grams to moles, converting the volume of gas in liters to moles uses a simple conversion formula. To calculate molarity, you can start with moles and volume, mass and volume, or moles and milliliters. Plugging these variables into the basic formula for calculating molarity will give you the correct answer. The volume and temperature are linearly related for 1 mole of methane gas at a constant pressure of 1 atm.
If the temperature is in kelvin, volume and temperature are directly proportional. Charles's law states that the volume of a given amount of gas is directly proportional to its temperature on the kelvin scale when the pressure is held constant. This relationship shows us that if we increase the moles of gas, n, by adding more gas while maintaining the same temperature and pressure, the volume of gas, V, will also increase. Molarity is not the same as concentration, although they are very similar.
Concentration is a measure of how many moles of a substance are dissolved in an amount of liquid, and can have any volume units. Molarity is a type of concentration, specifically moles per liter of solution. This means equal amounts of moles of gases occupy the same volume under the same conditions of temperature and pressure. Concentrations are often reported on a mass-to-mass (m/m) basis or on a mass-to-volume (m/v) basis, particularly in clinical laboratories and engineering applications.
A concentration expressed on an m/m basis is equal to the number of grams of solute per gram of solution; a concentration on an m/v basis is the number of grams of solute per milliliter of solution. Each measurement can be expressed as a percentage by multiplying the ratio by 100; the result is reported as percent m/m or percent m/v. For aqueous solutions at 20°C, 1 ppm corresponds to 1 μg per milliliter, and 1 ppb corresponds to 1 ng per milliliter.
These concentrations and their units are summarized in Table 4.1 "Common Units of Concentration". In Part A, you will time yourself counting popcorn kernels and figure out how many years it would take to count a mole of kernels. In Part B, you will measure the mass of a dozen popcorn kernels and a dozen marbles.